... eyes

The positions of the eyes has been determined by hand labelling on this figure 4.2

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... is

The particular case demonstrated above computes the probability of having the event "the next toss is a head" given that we have observed the sequence of event where all the 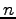$n$ toss observed were heads. Thus we have 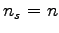$n_s=n$ because all the toss observed were heads, 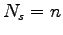$N_s=n$ because 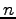$n$ toss were observed and 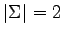$\vert\Sigma\vert=2$ because there were 2 possible events for each toss : "the toss is a head" and "the toss is a tail".

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.

... 3

note that the depth of trees learn in section 6.2 was limited to four in order to limit the computational cost

.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.
.