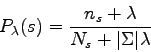
The Lidstone's law of succession is given by:
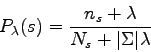
where the parameter ![]() is in the range
is in the range
![]() . It has been shown that this class of probability estimates is in fact a linear interpolation between the maximum likelihood estimate given in section 6.3.3.2 and the uniform prior
. It has been shown that this class of probability estimates is in fact a linear interpolation between the maximum likelihood estimate given in section 6.3.3.2 and the uniform prior
![]() . Indeed, we can define a new constant
. Indeed, we can define a new constant ![]() by:
by:
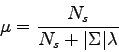
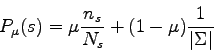
It is interesting to consider particular cases of the Lidstone's law of succession:
![]() thus represents the trust we have in relative frequencies.
thus represents the trust we have in relative frequencies. ![]() implies more trust in relative frequencies than the Laplace's law of succession while
implies more trust in relative frequencies than the Laplace's law of succession while ![]() represents less trust in relative frequencies. In practice, people use values of
represents less trust in relative frequencies. In practice, people use values of ![]() in the range
in the range
![]() , a common value being
, a common value being
![]() [81].
[81].